2.5 — Contestable Markets
ECON 316 • Game Theory • Fall 2021
Ryan Safner
Assistant Professor of Economics
safner@hood.edu
ryansafner/gameF21
gameF21.classes.ryansafner.com
Contestable Markets
Is Monopoly a Nash Equilibrium?
Now that we understand Nash equilibrium and the economics of oligopoly...
Are outcomes of other market structures Nash equilibria?


Is Monopoly a Nash Equilibrium?
Now that we understand Nash equilibrium and the economics of oligopoly...
Are outcomes of other market structures Nash equilibria?
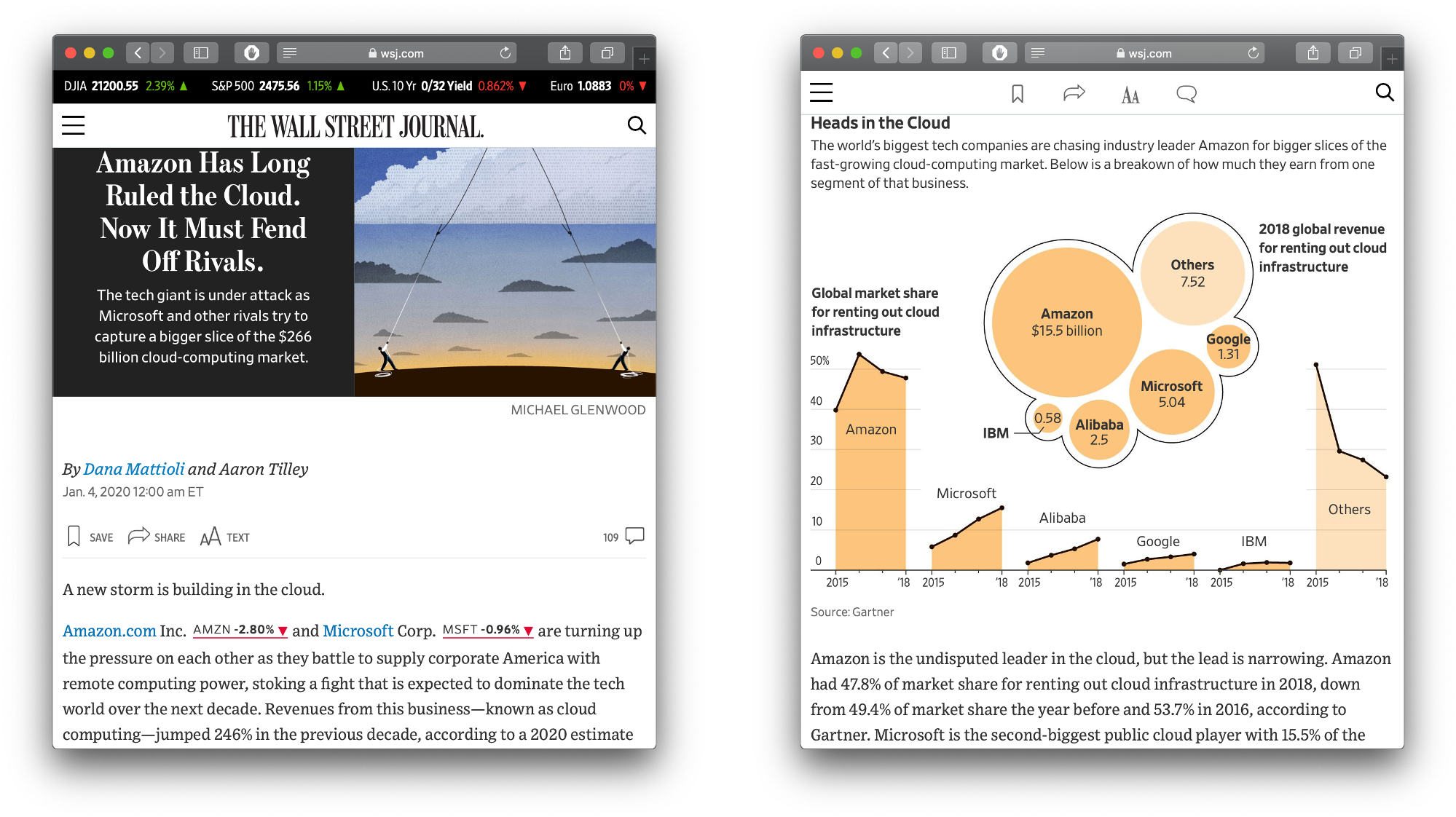
Perfect competition: no firm wants to raise or lower price given the market price ✓


Is Monopoly a Nash Equilibrium?
Monopolist maximizes π by setting q∗: MR=MC and p∗=Demand(q∗)
This is an equilibrium, but is it the only equilibrium?
We've assumed just a single player in the model
What about potential competition?


An Entry Game
- Model the market as an entry game, with two players:
Incumbent which sets its price pI
Entrant decides to stay out or enter the market, setting its price pE
- Bertrand (price) competition between 2 firms with similar products ⟹ consumers buy only from firm with lower price


Scenario I: Identical Costs
Contestable Markets II
Suppose both firms have identical costs: C(q)=cqMC(q)=c
If Incumbent sets pI>c
- Entrant would enter and set pE=pI−ϵ†

† For arbitrary ϵ>0, think ϵ= “one penny”
Contestable Markets II
Suppose both firms have identical costs: C(q)=cqMC(q)=c
If Incumbent sets pI>c
- Entrant would enter and set pE=pI−ϵ†
- Incumbent foresees this possibility, and wants to lower its price pI<pE
- This potential undercutting would continue logically until...

† For arbitrary ϵ>0, think ϵ= “one penny”
Contestable Markets II
Nash Equilibrium: (pI=c, Stay Out )
A market with a single firm, but the competitive outcome!
- p∗=MC, π=0
- competitive q∗
- max Consumer Surplus, no DWL
Scenario II: Entrant has Higher Costs
Contestable Markets II
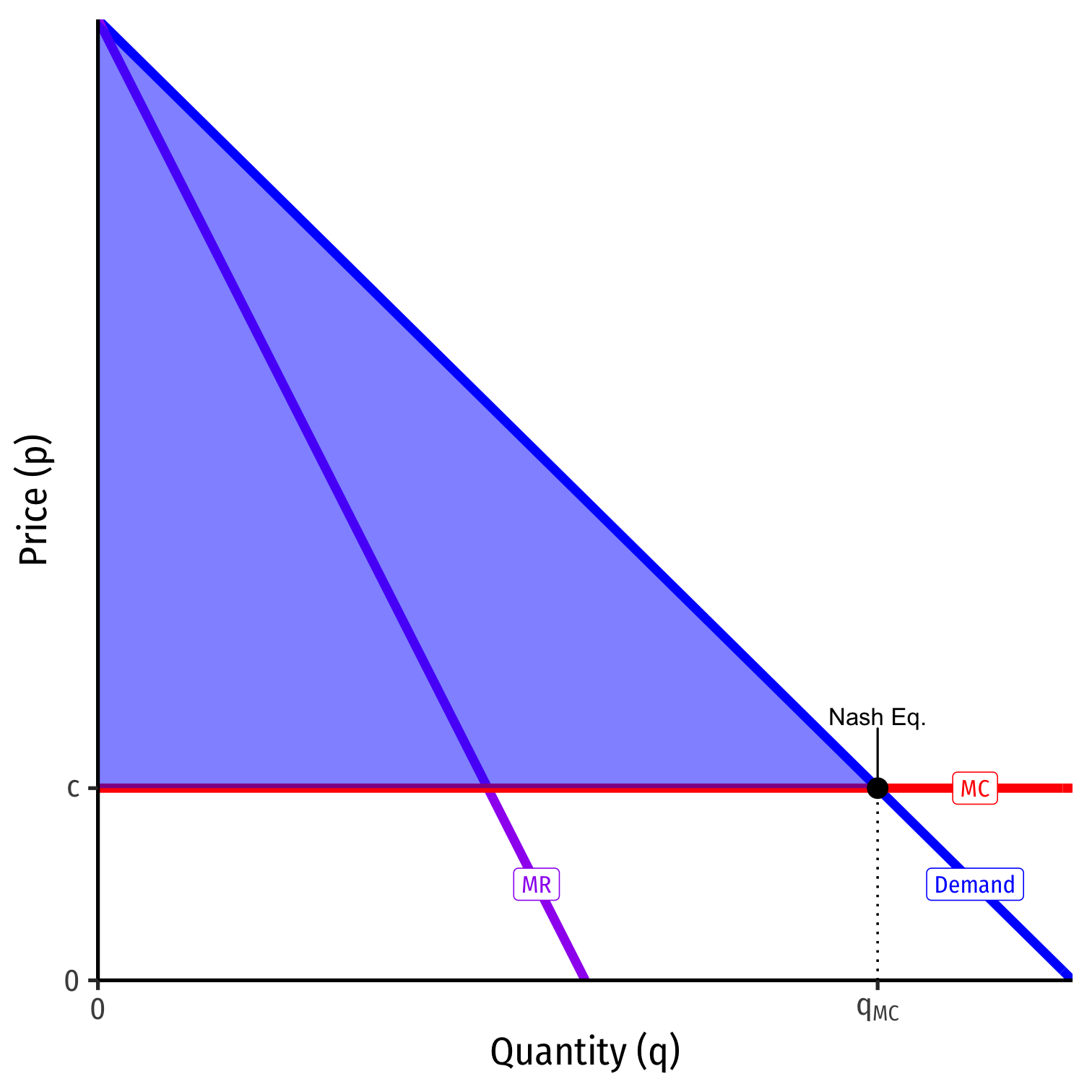
- What if the Entrant has higher costs than the Incumbent: cE>cI?
Contestable Markets II
What if the Entrant has higher costs than the Incumbent: cE>cI?
Nash equilibrium: (pI=pE−ϵ, Stay Out )
One firm again, with some inefficiency
- But not as bad as monopoly!
Scenario III: Fixed Costs
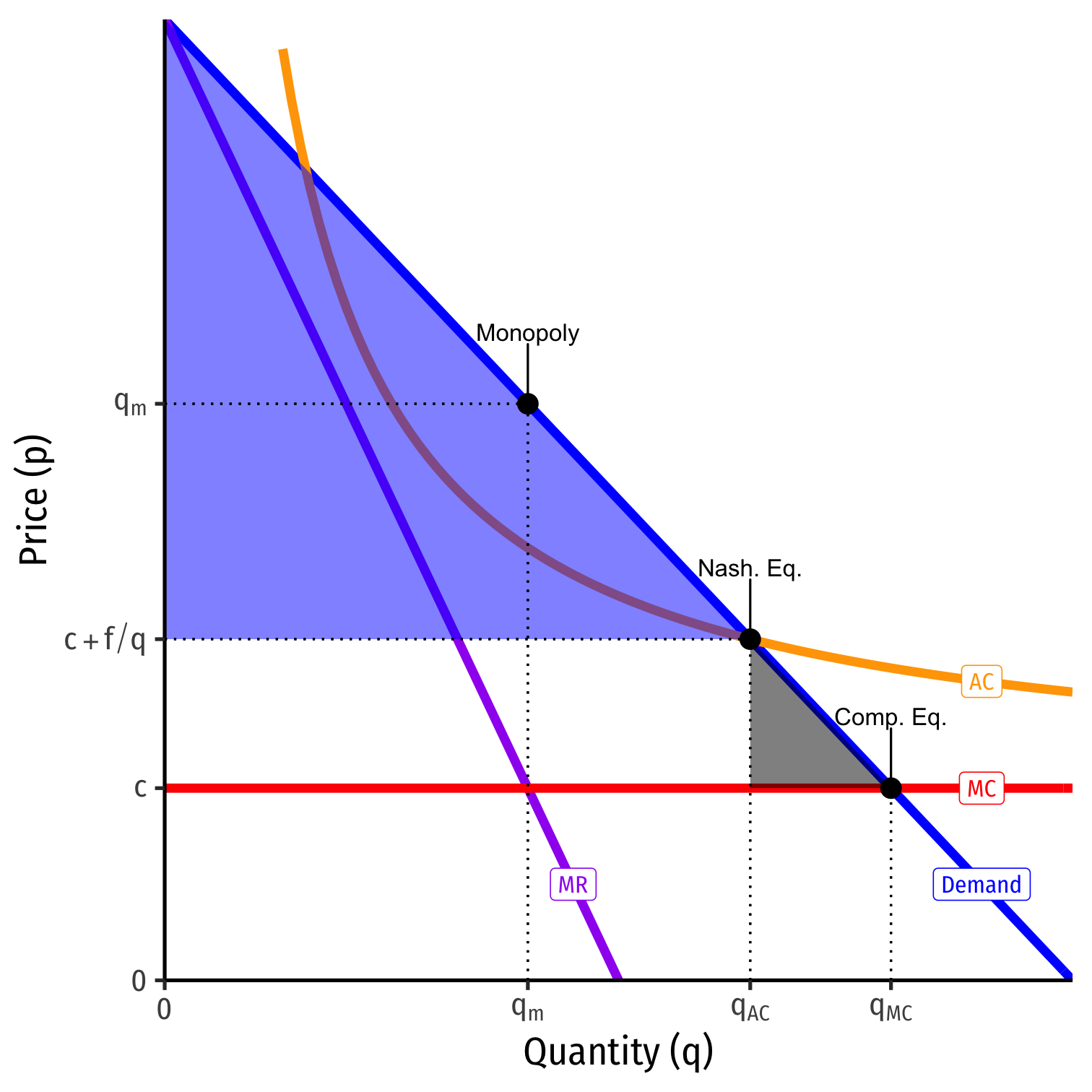
Contestable Markets III
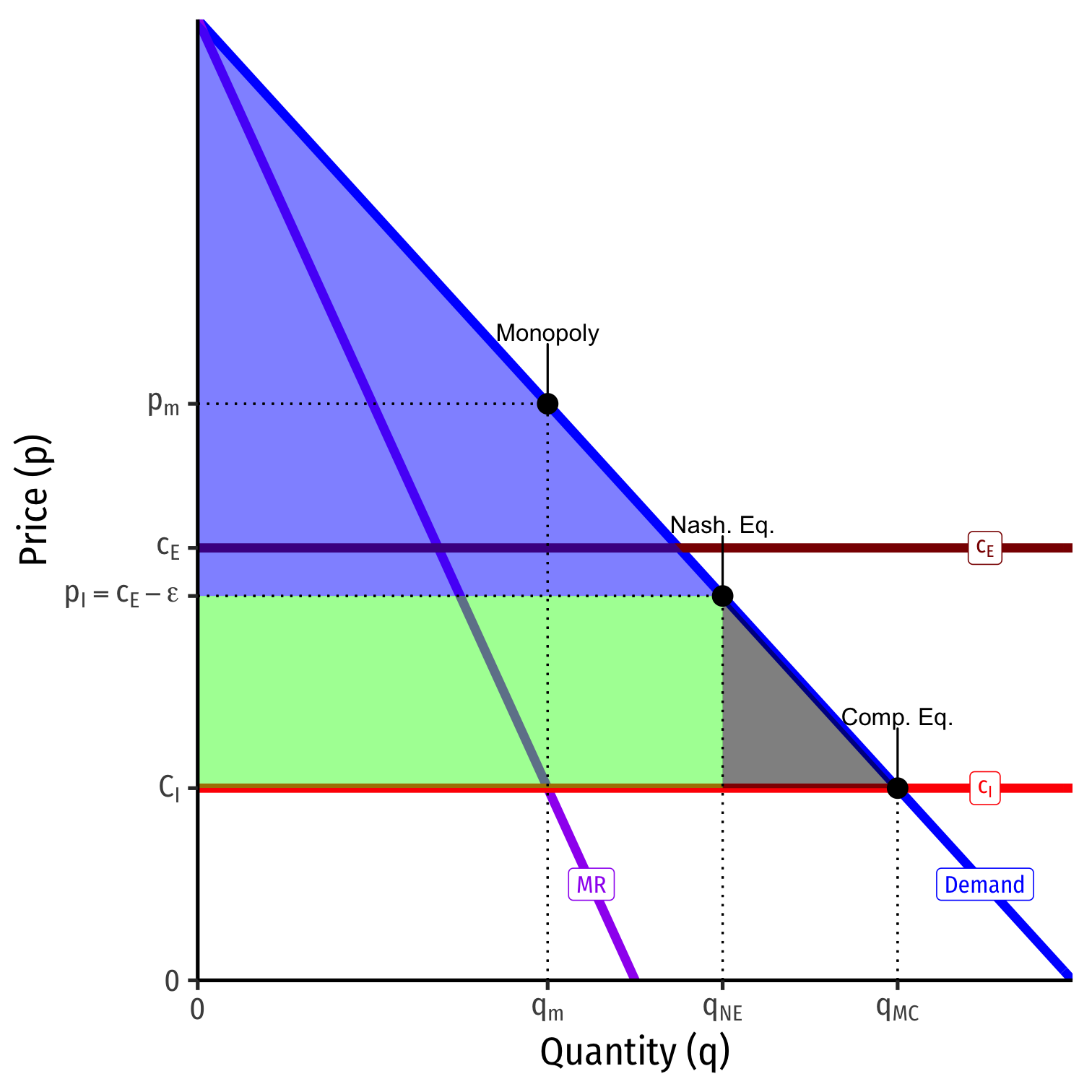
- What if there are fixed costs, f?
C(q)=cq+fMC(q)=cAC(q)=c+fq
- With high enough f, economies of scale prevent marginal cost pricing from a being profitable Nash Equilibrium
πp=MC=−fq<0
Contestable Markets IV
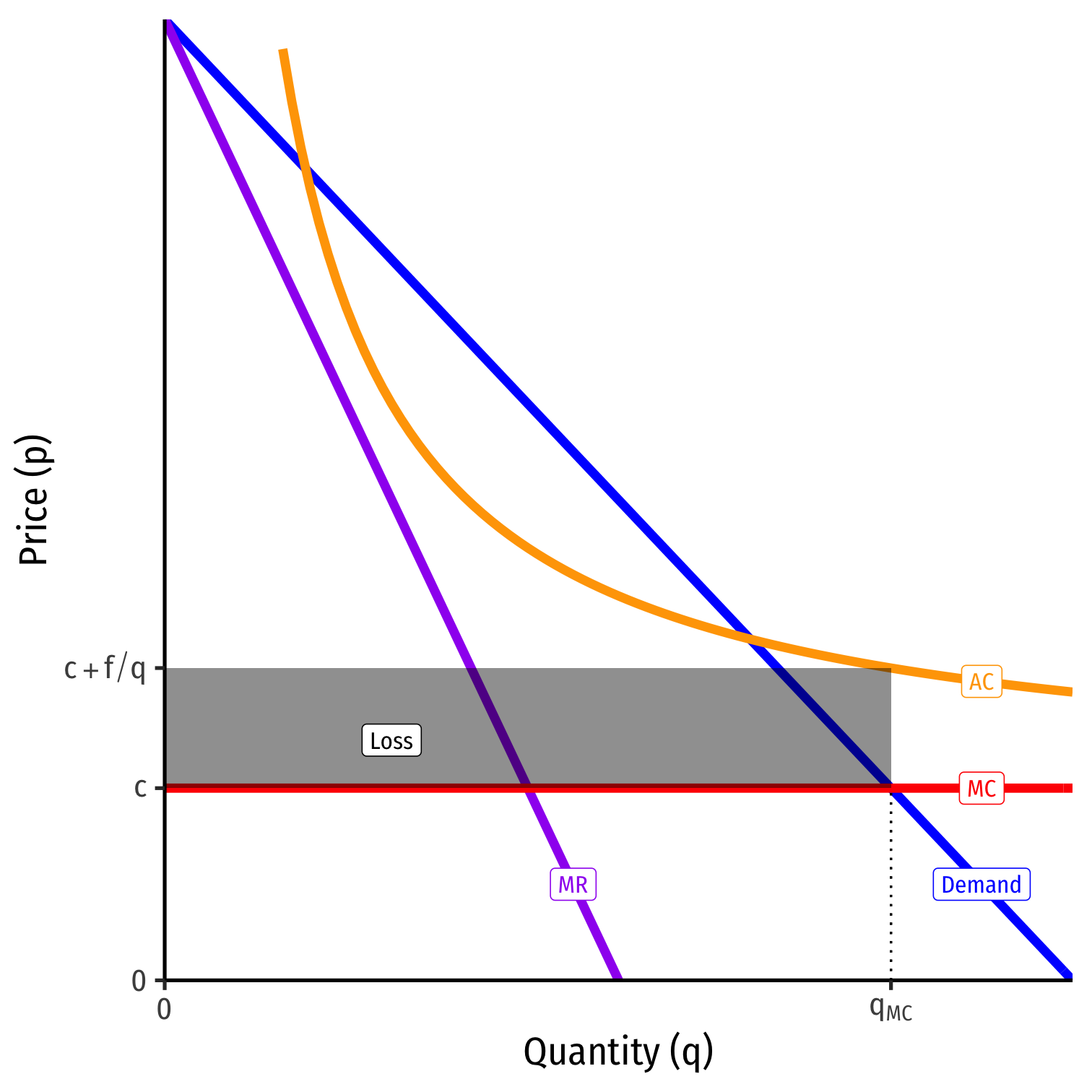
Nash equilibrium: (pI=AC, Stay Out )
Again, only a single firm with some inefficiency
- But not as bad as monopoly!
- Incumbent earns no profits!
Scenario IV: Sunk Costs
What About Sunk Costs? I
Fixed costs ⟹ do not vary with output
If firm exits, could sell these assets (e.g. machines, real estate) to recover costs
- Thus, “hit-and-run” competition remains potentially profitable
- Maintains credible threat against incumbent acting as a monopolist

What About Sunk Costs? I
But what if assets are not sellable and costs not recoverable - i.e. sunk costs?
e.g. research and development, spending to build brand equity, advertising, worker-training for industry-specific skills, etc

What About Sunk Costs? II
These are bygones to the Incumbent, who has already committed to producing
But are new costs and risk to Entrant, lowering expected profits
In effect, sunk costs raise cE>cI, and return us back to our Scenario II
Nash equilibrium: Incumbent deters entry with pI=pE−ϵ
- Inefficient, p>AC, but again not monopoly

Contestable Markets: Recap
Markets are contestable if:
- There are no barriers to entry or exit
- Firms have similar technologies (i.e. similar cost structure)
- There are no sunk costs
Economies of scale need not be inconsistent with competitive markets (as is assumed) if they are contestable
Generalizes “perfect competition” model in more realistic way, also game-theoretic

Contestable Markets: Summary

William Baumol
(1922--2017)
"This means that...an incumbent, even if he can threaten retaliation after entry, dare not offer profit-making opportunities to potential entrants because an entering firm can hit and run, gathering in the available profits and departing when the going gets rough."
Baumol, William, J, 1982, "Contestable Markets: An Uprising in the Theory of Industry Structure," American Economic Review, 72(1): 1-15
Implications for Markets and Policy
Implications for Competition

Regulation & antitrust (once) focus(ed) on number of firms
- "Count the number of firms, if it's 1, it's a monopoly!"
Perfect competition as “gold standard”, only market arrangement that is socially efficient:
- Allocatively efficient: p=MC, DWL=0
- Productively efficient: p=ACmin
Implications for Competition

But number of firms is endogenous and can evolve over time!
- Function of how firms mutually interact strategically
A more dynamic situation: firms respond over time
Implications for Competition

Perfect competition not the only socially efficient market-structure
- Market with number of firms (even 1) may be efficient if it is contestable
Regulation and antitrust should consider whether a market is contestable, not just the number of firms
- Free entry
- No sunk costs
Implications for Competition

Firms engaging in egregious monopolistic behavior (↓q, ↑p>MC, π>0) largely persist because of barriers to entry
- Attempts to make market uncontestable
Business activities or political dealings with the goal to raise cE>cI
- Lower your own costs, or raise your rivals'!
- (Recall Cournot competition with different costs)
Monopoly Or Contestable Market?

Contestable Markets
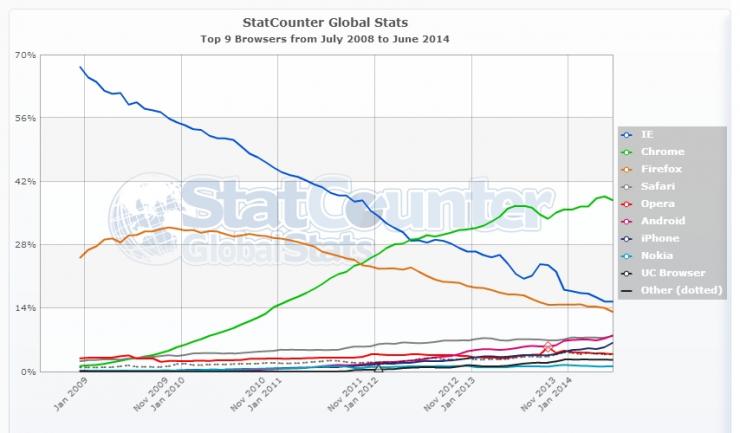
"Of far greater concern to Microsoft is the competition from new and emerging technologies, some of which are currently visible and others of which certainly are not. This array of known, emerging, and wholly unknown competitors places enormous pressure on Microsoft to price competitively and innovate aggressively." (Schmalensee 1999)
Contestable Markets
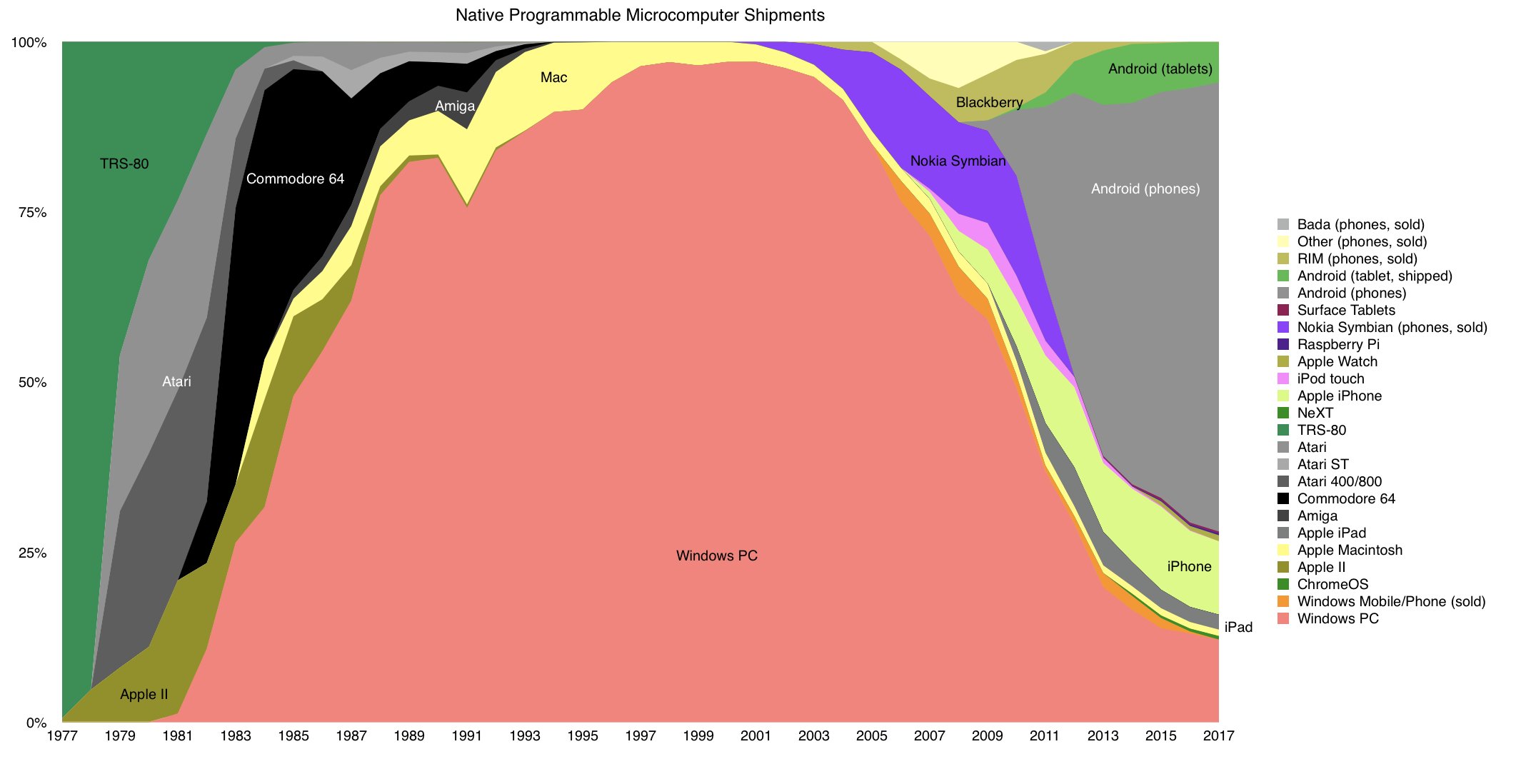
Contestable Markets