Question 1

What is the difference between a game with imperfect information and incomplete information?

Question 2

Describe the conditions for a Bayesian Nash Equilibrium (BNE) and Perfect Bayesian Nash Equilibrium (PBNE). Explain the two types of potential equilibria in any Bayesian game.

Question 3

Explain the framework of a signaling game. What kind of equilibrium is a good signal seeking to attain? (Think back to question 2.) What makes for a good signal? Give some examples.

Question 4

A group of police officers have breathalyzers that never fail to detect a truly drunk person. However, the breathalyzer displays false drunkenness in 5% of the cases in which the driver is sober. One in a thousand drivers is driving drunk.

Suppose the police officers stop a driver at random, and force the driver to take a breathalyzer test. It indicates that the driver is drunk. Assume we don’t know anything else; what is the probability the driver truly is drunk?

Hint: you will first need to find the probability of a positive result overall, use the law of total probability: \[P(B)=P(B|A)P(A)+P(B|\text{Not }A)P(\text{Not }A)\]

Drawing a probability tree may also help visualize.

Question 5

Consider the following game: A (partially-drunk) Local is at a bar in the Old West, potentially looking to pick a fight. A Stranger comes to the bar for breakfast. The Stranger is either Tough or Weak, and the Local does not know which. Suppose either type is equally likely (i.e. \(p=0.50)\). If the Local picks a Fight, the Stranger earns a payoff of 2, and if the Local chooses to Ignore, the Stranger earns 4. The Local earns 0 from Ignoring, 2 from Fighting a weak Stranger, and -1 against a tough Stranger. The Stranger first decides on breakfast: Quiche (at a cost of 0), or Beer, which costs 1 for a tough and 3 for a weak Stranger. The game is set up in the tree below.

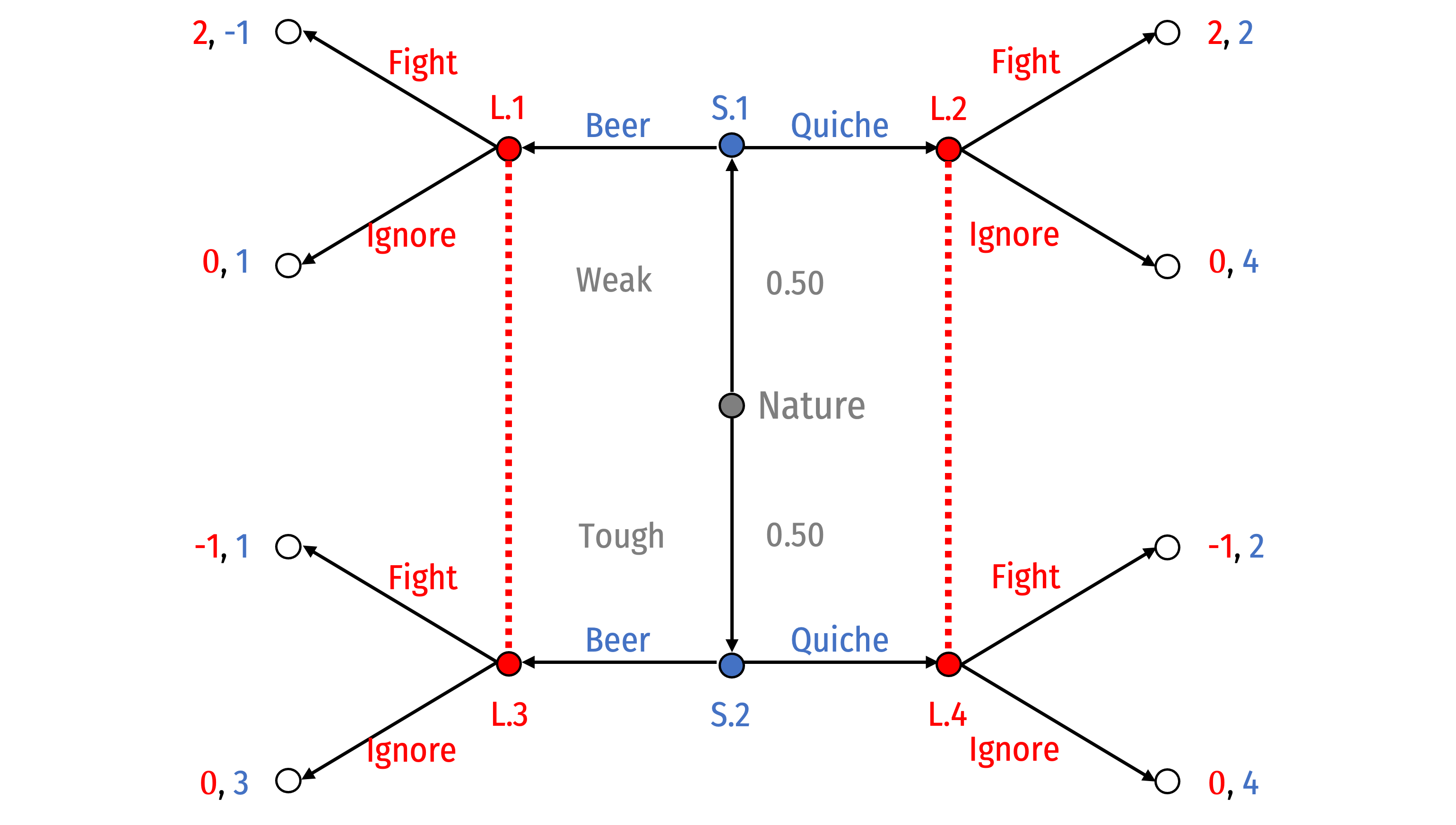
For the questions below, when contemplating a potential a Bayesian Perfect Nash Equilibrium (BPNE), each player must (1) have sequentially rational strategies that consistent with backwards induction and (2) have consistent beliefs about the other player. As hints, for (1), consider Local‘s expected payoff to picking a strategy against the two types of Stranger given Stranger’s breakfast selection. For (2), no need to worry about Bayes’ Law here, just think about what beliefs Local must have about Stranger’s type, conditional on observing Stranger’s breakfast choice, in each of the 4 equilibria.